Difference between revisions of "Sigma"
| Line 12: | Line 12: | ||
</td> | </td> | ||
<td> | <td> | ||
| − | [[ | + | [[image:bellcurve.jpg]] |
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
Revision as of 23:39, 3 October 2011
|
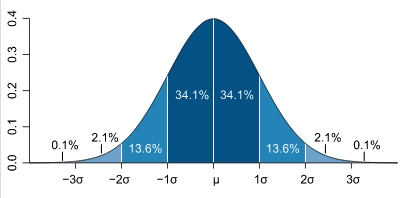
Most have heard of the "bell curve" in mathematics. This is the shape that describes the probability that a given percentage of measurements will fall in the region under the bell curve. Gausian Distribution simplified means that if you measure the same thing many times and make a vertical bar for each measurement value you can get, the bar graph will have a bell shape centered around the actual measurement. The width compared to the height of the bell shaped graph can be described with a statistical measurement called standard deviation or sigma. Basically, this is a measurement of how much the measurements vary around the actual value. Now three sigma is 3* the standard deviation, which statistically mean that 99.73% of the time a measurement is made it will be within 3*the standard deviation of the actual value. It is thus a way to compare how good the measurement method is. In similar ways 2 sigma means within 95% of the actual value and 6 sigma means as close to always as is resonable to ever need. |