Difference between revisions of "Sigma"
(→HOW SIGMA PERTAINS to the BELL CURVE) |
|||
| Line 15: | Line 15: | ||
===HOW SIGMA PERTAINS to the BELL CURVE=== | ===HOW SIGMA PERTAINS to the BELL CURVE=== | ||
| − | A statistical measurement called "standard deviation" is also referred to as "sigma" on the horizontal axis of a bell curve. Basically, this is a measurement of how much the measurements vary | + | A statistical measurement called "standard deviation" is also referred to as "sigma" on the horizontal axis of a bell curve. Basically, this is a measurement of how much the measurements vary from the center value by a distance of a value called "sigma" on either side of the center of the curve. |
| + | === 1 SIGMA === | ||
| − | + | If someone says that an error is 1 sigma, then that person is referring to the greatest possible error in between the lines marked with -1 and +1 sigma in the bell curve (see the curve above). | |
| − | If someone says that | + | |
Now three sigma is 3* the standard deviation, which statistically mean that 99.73% of the time a measurement is made it will be within 3*the standard deviation of the actual value. It is thus a way to compare how good the measurement method is. | Now three sigma is 3* the standard deviation, which statistically mean that 99.73% of the time a measurement is made it will be within 3*the standard deviation of the actual value. It is thus a way to compare how good the measurement method is. | ||
In similar ways 2 sigma means within 95% of the actual value and 6 sigma means as close to always as is resonable to ever need. | In similar ways 2 sigma means within 95% of the actual value and 6 sigma means as close to always as is resonable to ever need. | ||
Revision as of 23:51, 3 October 2011
|
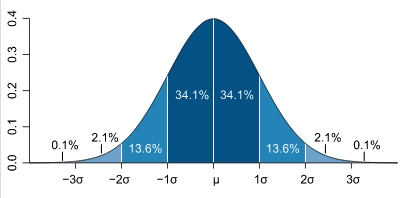
Most have heard of the "bell curve" in mathematics. This is the shape that describes the probability that a given percentage of measurements will fall within a certain region under a standard bell curve. This shape is found in nature - so its one that mathematicians use often. This shape of the standard bell curve is called "Normal Distribution" or "Gausian Distribution." |
HOW SIGMA PERTAINS to the BELL CURVE
A statistical measurement called "standard deviation" is also referred to as "sigma" on the horizontal axis of a bell curve. Basically, this is a measurement of how much the measurements vary from the center value by a distance of a value called "sigma" on either side of the center of the curve.
1 SIGMA
If someone says that an error is 1 sigma, then that person is referring to the greatest possible error in between the lines marked with -1 and +1 sigma in the bell curve (see the curve above).
Now three sigma is 3* the standard deviation, which statistically mean that 99.73% of the time a measurement is made it will be within 3*the standard deviation of the actual value. It is thus a way to compare how good the measurement method is.
In similar ways 2 sigma means within 95% of the actual value and 6 sigma means as close to always as is resonable to ever need.